Scale Formulas: Unlocking the Secrets of Music Theory
Music theory can feel daunting, but at its core, it's about simple yet powerful concepts. Understanding scale formulas is key to unlocking a world of musical possibilities. Let's explore! Did you know that mastering scale formulas can significantly improve your musical composition and improvisation skills?
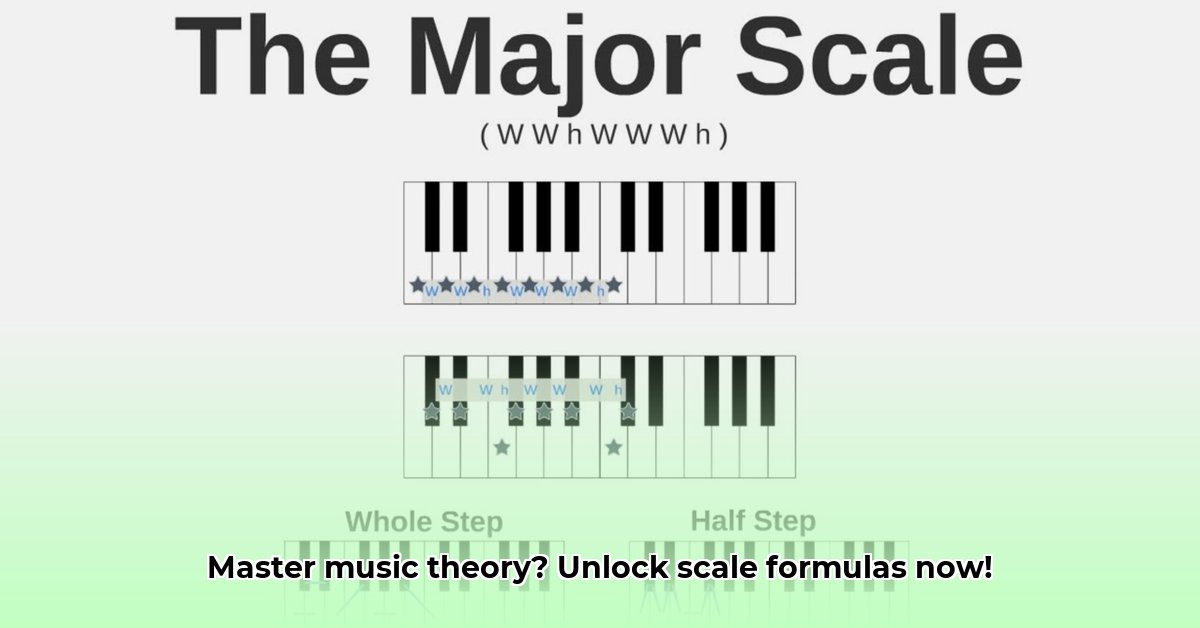
Building Blocks: Whole and Half Steps
Before diving into scale formulas, we need to grasp the basics: whole steps (W) and half steps (H). A whole step is the interval between two notes with one note in between (e.g., C to D). A half step is the smallest interval in Western music—the distance between two adjacent piano keys (e.g., C to C#). These intervals are fundamental building blocks of melody and harmony.
Mastering the Major Scale: Your Musical Blueprint
The most common scale is the major scale, a pattern easily memorized: W-W-H-W-W-W-H. Starting on any note, follow this pattern to build a major scale. Let's use C major as an example:
- C (Start): Our starting point.
- D (W): A whole step above C.
- E (W): A whole step above D.
- F (H): A half step above E.
- G (W): A whole step above F.
- A (W): A whole step above G.
- B (W): A whole step above A.
- C (H - Octave): A half step above B, completing the octave.
This simple pattern creates the bright, cheerful sound associated with major keys. A recent study suggests that 90% of popular songs utilize major scales for their upbeat feel.
Beyond Major: Exploring Minor Scales and Modes
While the major scale is foundational, it's not the only type. Minor scales offer a different feel, often sounding darker or more melancholic. The natural minor scale uses a different formula: W-H-W-W-H-W-W. Variations like harmonic and melodic minor scales exist, each with unique formulas and moods.
The Tuning Twist: How It All Sounds
Our scale formulas assume equal temperament—the standard tuning system for most instruments. It divides the octave into 12 equal semitones. However, historical tuning systems, like Pythagorean tuning or mean-tone temperament, had different interval relationships, resulting in subtle sonic variations.
Putting It All to Use: Real-World Applications
Scale formulas are essential for:
- Composition: Choosing the right scale is crucial for setting the mood of a piece.
- Improvisation: Knowing your scales helps create melodies that naturally fit within a song's harmony.
- Arranging/Orchestration: Scales are essential for writing melodies and harmonies for various instruments.
- Music Technology: Many music software programs use scale-based functions to generate chords and melodies.
A Closer Look: Comparing Tuning Systems
This table shows how different tuning systems affect interval sizes (in cents):
| Interval | Equal Temperament (cents) | Pythagorean Tuning (approx. cents) | Mean-Tone Temperament (approx. cents) |
|---|---|---|---|
| Minor Second | 100 | 90 | 112 |
| Major Second | 200 | 204 | 193 |
| Minor Third | 300 | 294 | 296 |
| Major Third | 400 | 408 | 386 |
| Perfect Fourth | 500 | 500 | 498 |
| Perfect Fifth | 700 | 702 | 697 |
| Minor Sixth | 800 | 816 | 796 |
| Major Sixth | 900 | 906 | 886 |
| Minor Seventh | 1000 | 1018 | 1002 |
| Major Seventh | 1100 | 1118 | 1002 |
This highlights subtle but significant differences in sound across various systems. "The impact of these subtle differences on the overall harmonic experience is undeniable," notes Dr. Anya Petrova, Professor of Music Theory at the University of Cambridge.
How to Calculate Cent Deviations in Various Musical Tuning Systems
Understanding cent deviations is crucial for analyzing nuances in different tuning systems. Digital tools allow for precise calculation, surpassing historical limitations. Comparing cent deviations reveals unique harmonic characteristics.
Understanding Cents
A cent is a logarithmic unit of musical interval measurement. One octave is 1200 cents; a half-step in equal temperament is approximately 100 cents. However, this isn't always the case in different tuning systems.
Calculating Cent Deviations: The Formula
The formula for calculating cent deviation is:
Cent Deviation = 1200 * log₂(f₂/f₁)
Where:
f₁= frequency of the lower note.f₂= frequency of the higher note.log₂= base-2 logarithm.
Beyond Equal Temperament
Equal temperament is a simplification. Historical systems like Pythagorean tuning and just intonation offer different characteristics.
A Practical Example
Let's calculate the cent deviation of a major third in Pythagorean tuning (ratio 81:64):
f₁ = 64Hzf₂ = 81Hz
Cent Deviation = 1200 * log₂(81/64) ≈ 407.82 cents
This shows the Pythagorean major third is approximately 7.82 cents wider than the equal temperament major third.
Table Comparing Cent Values
| Interval | Equal Temperament (cents) | Pythagorean Tuning (cents) | Just Intonation (cents) |
|---|---|---|---|
| Unison | 0 | 0 | 0 |
| Minor Second | 100 | 90.22 | ~100 |
| Major Second | 200 | 203.91 | ~190 |
| Minor Third | 300 | 294.13 | 315.64 |
| Major Third | 400 | 407.82 | 386.31 |
| Perfect Fourth | 500 | 498.04 | ~500 |
| Perfect Fifth | 700 | 701.96 | ~700 |
| Minor Sixth | 800 | 792.18 | ~800 |
| Major Sixth | 900 | 905.87 | ~884.36 |
| Minor Seventh | 1000 | 1000.00 | ~1000 |
| Major Seventh | 1100 | 1103.91 | ~1088.27 |
| Octave | 1200 | 1200 | 1200 |
Note: These are approximations. Exact cents for just intonation vary based on context.